Python: eigenvalues with Scipy and Sympy

Eigenvalues and eigenvectors are important in systems of differential equations. To speed up my analysis I decided to use some numerical package for getting eigenvalues form square matrices. I found two numerical packages Scipy and Sympy for Python. Both have ability to calculate eigenvalues. But their calculations are not reliable. I present few very simple examples showing how rubbish these packages are.

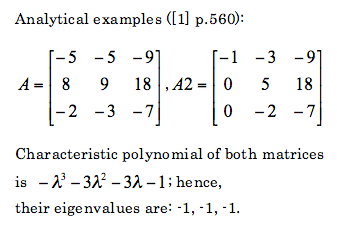
Lets see what does Python calculate:
Scipy
from scipy import *
from scipy.linalg import *

A=matrix([ [-5,-5,-9],[8,9,18],[-2,-3,-7] ])
print eigvals(A)
# [-0.999989 +1.90461984e-05j -0.999989 -1.90461984e-05j
# -1.00002199 +0.00000000e+00j]

A2=matrix([ [-5,-5,-9],[8,9,18],[-2,-3,-7] ])
print eigvals(A2)
# [-1.+0.j 　　-1.00000005+0.j 　　-0.99999995+0.j]
Sympy
from sympy import *
from sympy.matrices import Matrix
x=Symbol('x')
A=Matrix(( [-5,-5,-9],[8,9,18],[-2,-3,-7] ))
print roots(A.charpoly(x),x)
# Returns error!!!

A2=Matrix(( [-1,-3,-9],[0,5,18],[0,-2,-7] ))
print roots(A2.charpoly(x),x)
# Returns error!!!

Based on these to examples it is clearly seen that these two packaged are unreliable, at least as far as calculation of eigenvalues is considered.

For matrices 2x2 it was noticed that the eigenvalues are correctly calculated both for real and complex values.

For comparison lets check what does Matlab returns:A=[-5,-5,-9;8,9,18;-2,-3,-7]
eig(A)

ans =

-1.0000 + 0.0000i
-1.0000 - 0.0000i
-1.0000

A2=[-5,-5,-9;8,9,18;-2,-3,-7]
eig(A2)

ans =

-1.0000 + 0.0000i
-1.0000 - 0.0000i
-1.0000

So it is seen that Matlab does correctly calculate these eigenvalues.


Even if one manually calculates characteristic polynomial and would like to gets roots of it, I would recommend neither Scipy or Sympy. As an example I calculate roots of the previously obtained characteristic polynomial for matrices A and A2:
import scipy as sc
p=sc.poly1d([-1,-3,-3,-1])
print p= sc.roots(p)
# [-1.0000086 +0.00000000e+00j -0.9999957 +7.44736442e-06j
# -0.9999957 -7.44736442e-06j]
import sympy as sy
x=sy.Symbol('x')
print sy.solve(-x**3-3*x**2-3*x-1==0,x)
#[-1]

As can be seen calculation of roots of polynomials is also not good. In the second case there is one root (-1) instead of three (-1,-1,-1).

For comparison lets check Matlab (or Octave 3):p=[-1,-3,-3,-1]
roots(p)

ans =

-1.0000
-1.0000 + 0.0000i
-1.0000 - 0.0000i


Based on these results it is seen that its better to use Matlab for calculation of eignevalues.

References:
[1] S.I. Grossman, Multivariable calculus, linear algebra and differential equation, Second edition, 1986